Twisted Function
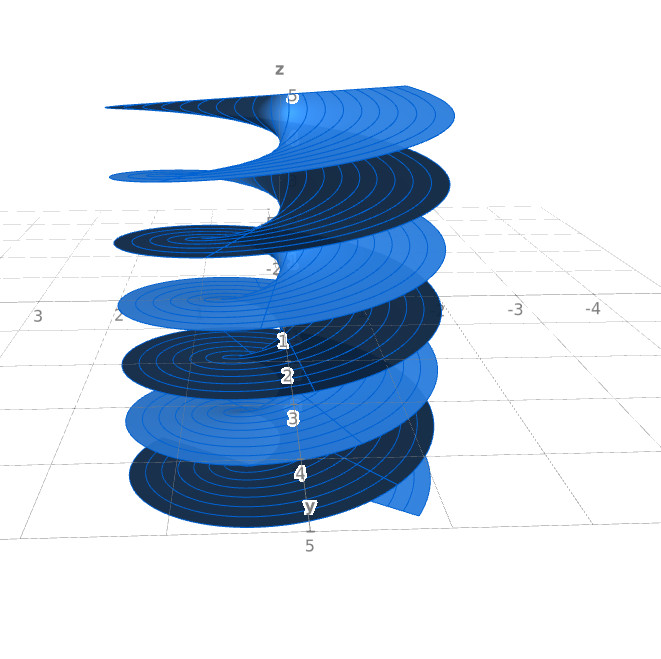
Function \(F(u,v) = (u \cdot \cos(v), u \cdot \sin(v), 0.5 v)\) maps \(\mathbb{R}^2\) to a surface called Helicoid. What is the inverse of this function? That is, provide a function \(F^{-1}(x,y,z)\) that takes a point on the helix surface and returns the corresponding point in \(\mathbb{R}^2\).
The inverse function of \(F\) is
equal to \(F^{-1}(x,y,z) = (y \cdot \sin(2z) +
x \cdot \cos(2z), 2z)\).
Since \(F(u,v) = (u \cdot \cos(v), u \cdot
\sin(v), 0.5 v)\), if we define \(F^{-1}(x,y,z) = (u,v)\) it implies that
\(v=2z\). To determine the value of
\(u\) we leverage the fact that \(\sin^2(v) + \cos^2(v) = 1\) which implies:
\[\begin{aligned}
u &= u \cdot (\sin^2(v) + \cos^2(v))\\
&= (u \cdot \sin(v)) \cdot \sin(v) + (u \cdot \cos(v)) \cdot \cos(v)
\\
&= y \cdot \sin(v) + x \cdot \cos(v) \\
&= y \cdot \sin(2z) + x \cdot \cos(2z).
\end{aligned}\]